Задание
В треугольнике \(\displaystyle ABC\) угол \(\displaystyle C\) равен \(\displaystyle 90^{\circ} {\small,}\) \(\displaystyle \tg \angle B=\frac{7}{12} {\small,}\) \(\displaystyle BC=48 {\small.}\) Найдите \(\displaystyle AC {\small.}\)
Решение
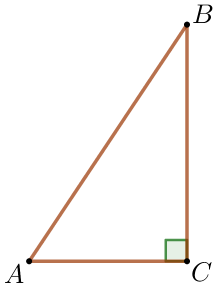
В прямоугольном треугольнике тангенс острого угла равен отношению противолежащего катета к прилежащему катету. Значит,
\(\displaystyle \tg \angle B= \frac{AC}{BC}{\small.}\)
По условию \(\displaystyle \tg \angle B=\frac{7}{12} {\small,}\) \(\displaystyle BC=48 {\small.}\) Тогда
\(\displaystyle \frac{7}{12}= \frac{AC}{48}{\small.}\)
Следовательно,
\(\displaystyle AC=\frac{7}{12} \cdot {48} =28{\small.}\)
Ответ: \(\displaystyle 28{\small.}\)