Человек стоит на расстоянии \(\displaystyle 2{,}2\)м от столба, на котором висит фонарь, расположенный на высоте \(\displaystyle 6\)м. Тень человека равна \(\displaystyle 0{,}8\)м. Какого роста человек (в метрах)?
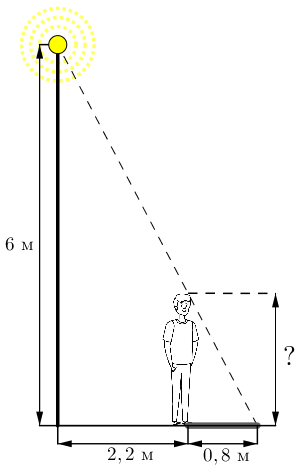
Пусть \(\displaystyle \red{ h}\) – рост человека.
Изобразим предложенную в задаче конструкцию в виде прямоугольных треугольников
\(\displaystyle \triangle ABC \) и \(\displaystyle \triangle DBH\small, \) в которых:
- точка \(\displaystyle D\) лежит на \(\displaystyle AB\small, \) точка \(\displaystyle H\) лежит на \(\displaystyle BC\small, \)
- \(\displaystyle \angle ACB =90^{\circ} \small, \) \(\displaystyle \angle DHB =90^{\circ} \small, \)
- \(\displaystyle CH= 2{,}2 \small, \) \(\displaystyle BH=0{,}8 \small, \) \(\displaystyle AC= 6 \small, \) \(\displaystyle DH=\red{h} \small. \)
Рассмотрим треугольники \(\displaystyle \triangle ABC \) и \(\displaystyle \triangle DBH\small. \)
Так как \(\displaystyle \angle ACB =\angle DHB =90^{\circ} \) и\(\displaystyle \angle B \) – общий,
то треугольники \(\displaystyle \triangle ABC \) и \(\displaystyle \triangle DBH\) подобны по двум углам.
Тогда \(\displaystyle \frac{AC}{DH}=\frac{BC}{BH} \small.\) Выразим \(\displaystyle DH\small: \) \(\displaystyle DH= \frac{AC \cdot BH}{BC} \small, \\ \) \(\displaystyle \red{h}= \frac{6 \cdot 0{,}8}{BC} \small.\) Найдем \(\displaystyle BC \small: \) \(\displaystyle BC=CH+BH \small,\) \(\displaystyle BC= 2{,}2+0{,}8=3 \small.\) Получаем \(\displaystyle \red{h}= \frac{6 \cdot 0{,}8}{3}=2 \cdot 0{,}8=1{,}6 \small.\) | 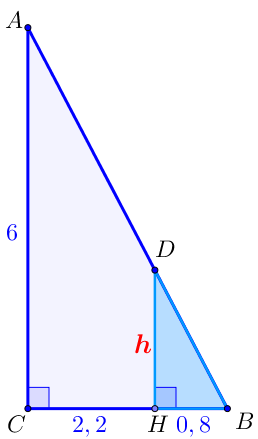 |
Рост человека равен \(\displaystyle 1{,}6\) метра.
Ответ: \(\displaystyle 1{,}6 \small. \)